Anode and Cathode Electrodes

Just realised today that anode and cathode need not be always + and -vely charged respectively.

Excerpt from a link:

http://www.av8n.com/physics/anode-cathode.htm
 Definition: The anode of a device is the terminal where current flows in from outside. The cathode of a device is the terminal where current flows out. This is illustrated in figure 1.

Figure 1: Anode and Cathode Currents

As always, electrons flowing in is the same as positive current flowing out, and vice versa.

http://en.wikipedia.org/wiki/Anode

http://en.wikipedia.org/wiki/Cathode

A widespread misconception is that anode polarity is always positive (+). This is often incorrectly inferred from the correct fact that in all electrochemical devices negatively charged anions move towards the anode (hence their name) and/or positively charged cations move away from it. In fact anode polarity depends on the device type, and sometimes even in which mode it operates, as per the above electric current direction-based universal definition. Consequently, as can be seen from the following examples, in a device which consumes power the anode is positive, and in a device which provides power the anode is negative:

 When the current through the device is reversed, the electrodes switch functions, so anode becomes cathode, while cathode becomes anode, as long as the reversed current is applied, with the exception of diodes where electrode naming is always based on the forward current direction.

Materials and Components

1. Components incapable of controlling current by means of another electrical signal are called passive devices. Resistors, capacitors, inductors, transformers, and even diodes are all considered passive devices. Active devices include, but are not limited to, vacuum tubes, transistors, silicon-controlled rectifiers (SCRs), and TRIACs.


http://www.allaboutcircuits.com/vol_3/chpt_1/2.html


2. Thyratron is an active device, a type of gas filled tube used as a high energy electrical switch and controlled rectifier.Unlike a vacuum tube, a thyratron cannot be used to amplify signals linearly.A thyratron is basically a "controlled gas rectifier".Gases used include mercuryvapor, xenon, neon, and (in special high-voltage applications or applications requiring very short switching times) hydrogen.


http://en.wikipedia.org/wiki/Thyratron

3. A reflex klystron oscillator is preferred as an ideal source in micro measurements as it provides low power output with multiple frequency components.The interaction between the electron beam and the radio frequency wave in the re-entrant cavity depends upon the average gap transit angle, this is the phase change due to finite time, the electron beam takes to cross the central gap of the re-entrant cavity.

http://www.tpub.com/neets/book11/45d.htm
http://www.worldlingo.com/ma/enwiki/en/Klystron

4. A step recovery diode is also known as snap-off varactor.

5. Schottky diode is a unipolar device because it has electrons as majority carriers on both sides of the junction.It can be used for rectification of signals of frequencies even exceeding 300 MHz. It is also used in clipping and clamping circuits and in computer gating. Its low noise figure finds application in sensitive applications like radars.

-Electronic devices and circuits, by Salivahanan




6. The graded index optical fibre cable can be considered to have smaller  
     numerical aperture than step index cable.




7. There are about 25 superconducting metallic elements at low temperatures, 
    like tin and aluminium.All superconductors have exactly zero resistivity to low 
     applied currents when there is no magnetic field present.Conventional superconductors
    usually have critical temperatures ranging from less than 1K to around 20K.


    When a superconductor is placed in a weak external magnetic field H, the field penetrates for
    only a short distance λ, called the penetration depth, after which it decays rapidly to zero. 
    This is called the Meissner effect. For most superconductors, the penetration depth is 
     on the order of a hundred nm. 
http://fy.chalmers.se/~delsing/LowTemp/LectureSC/Definition%20of%20SC.pdf




8. Porcelain is used as an insulator.Porcelain insulators are made from clay, 
   quartz or alumina and feldspar, and are covered with a smooth glaze to shed 
    water. Glass has a higher dielectric strength than porcelain.
http://en.wikipedia.org/wiki/Insulator_(electrical)

General Awareness -1

1. Oral Polio Vaccine (OPV):

http://en.wikipedia.org/wiki/Polio_vaccine
http://india.gov.in/citizen/health/faq_polio.php


Two polio vaccines are used throughout the world to combat poliomyelitis (or polio). The first was developed by Jonas Salk at the University of Pittsburgh and first tested in 1952. Announced to the world by Salk on April 12, 1955, it consists of an injected dose of inactivated (dead) poliovirus. An oral vaccine was developed by Albert Sabin using attenuated poliovirus. Human trials of Sabin's vaccine began in 1957 and it was licensed in 1962.


Every child during the first year of life should receive at least three routine doses of Oral Polio Vaccine (OVP). Interruption of person to person transmission of the virus by vaccination is the critical step in global polio eradication.OPV drops may also be given to newborn children, even if they were born only a few hours ago. Usually, the colour of an Oral Polio Vaccine (OPV) is pink. However, sometimes the colour may also be yellow or white. All the vaccines are the same and this colour difference in no way affects the quality or type of vaccine.




2.  Pineal Gland:

http://en.wikipedia.org/wiki/Pineal_gland
http://www.wayfinding.net/pineal.htm


It is a small endocrine gland in the vertebrate brain.It produces a hormone that affects the modulation of wake/sleep patterns and seasonal functions. It is located near the center of the brain between the two hemispheres.Chemical analysis shows that they are composed of calcium phosphate, calcium carbonate, magnesium phosphate, and ammonium phosphate.  

The pineal gland was called the "third eye" by ancient people. It was thought to have mystical powers. René Descartes, who dedicated much time to the study of the pineal gland,^[25] called it the "seat of the soul".^[26] He believed that it was the point of connection between the intellect and the body.

FET Concepts

http://en.wikipedia.org/wiki/Field-effect_transistor

1. The drain and source may be doped of opposite type to the channel, in the case of depletion mode FETs, or doped of similar type to the channel as in enhancement mode FETs.


2. The JFET (Junction Field-Effect Transistor) uses a reverse biased p-n junction to separate the gate from the body.


3. The MOSFET (Metal–Oxide–Semiconductor Field-Effect Transistor) utilizes an insulator (typically SiO₂) between the gate and the body.


4. The most commonly used FET is the MOSFET. The CMOS (complementary-symmetry metal oxide semiconductor) process technology is the basis for modern digital integrated circuits. This process technology uses an arrangement where the (usually "enhancement-mode") p-channel MOSFET and n-channel MOSFET are connected in series such that when one is on, the other is off.


5. 

http://www-ferp.ucsd.edu/najmabadi/CLASS/ECE60L/02-S/NOTES/FET.pdf 


6. A FET is a "voltage-controlled" device.


7. Complementary MOS technology employs MOS transistors of both polarites PMOS and NMOS, thus many powerful circuits are possible using CMOS.


8. Depletion-type MOSFET has a physically implanted channel. Thus, a n-type depletion-type MOSFET has already a n-type channel between drain and source.


http://www.bcae1.com/tranfet.htm. 


9


 


Schematic symbol for N channel MOSFET  


10.  

The transistor's "turn on" (a.k.a. threshold) voltage varies from one FET to another but is approximately 3.3 volts with respect to the source.

Complex Variables

Reference: http://www.math.uiuc.edu/~r-ash/CV/CV1.pdf
http://en.wikipedia.org/wiki/Cauchy%E2%80%93Riemann_equations
http://mathworld.wolfram.com/Cauchy-RiemannEquations.html


1. If z = a + bi, then a is called the real part of z, written a = R ez, and b is called the
imaginary part of z, written b = Imz. The absolute value or magnitude or modulus of z
is defined as (a2 +b2)1/2.


2. A complex number with magnitude 1 is said to be unimodular.

3. An argument of z (written arg z) is defined as the angle which the line segment from (0, 0)
to (a, b) makes with the positive real axis.

4. to a multiple of 2π.
If r is the magnitude of z and θ is an argument of z, we may write
z = r(cos θ + i sin θ)

5. The Cauchy–Riemann equations on a pair of real-valued functions u(x,y) and v(x,y) are the two equations:

(1a)     

and

(1b)    

Typically u and v are taken to be the real and imaginary parts respectively of a complex-valued function f(x + iy) = u(x,y) + iv(x,y). 

6. If 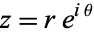, then the Cauchy-Riemann equations become

			(18)

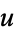7.  If  and 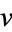 satisfy the Cauchy-Riemann equations, they also satisfy Laplace's equation in two dimensions, since

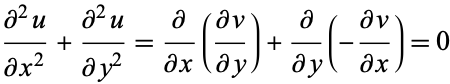(20)

8.  An analytic signal is a signal with no negative-frequency components 9.  A function is analytic if and only if it is equal to its Taylor series in someneighborhood of every point. 10. Any polynomial (real or complex) is an analytic function. This is because if a polynomial has degree n, any terms of degree larger than nin its Taylor series expansion will vanish, and so this series will be trivially convergent. Furthermore, every polynomial is its own Maclaurin series. 11.  The exponential function is analytic. Any Taylor series for this function converges not only for x close enough to x0 (as in the definition) but for all values of x (real or complex). The trigonometric functions, logarithm, and the power functions are analytic on any open set of their domain. Typical examples of functions that are not analytic are: The absolute value function when defined on the set of real numbers or complex numbers is not everywhere analytic because it is not differentiable at 0. Piecewise defined functions (functions given by different formulas in different regions) are typically not analytic where the pieces meet. The complex conjugate function  is not complex analytic, although its restriction to the real line is the identity function and therefore real analytic. 12. In mathematics, the Laurent series of a complex function f(z) is a representation of that function as a power series which includes terms of negative degree. It may be used to express complex functions in cases where a Taylor series expansion cannot be applied.

Verbal Analgoies

Verbal Analogies Practice Test